Remember that nerve-wracking feeling before a big math test? You’ve studied, you’ve practiced, but you still worry about those tricky transformations. Fear not, fellow learners! Mastering the concept of parent functions and transformations is the key to unlocking a whole new level of understanding in math. This guide will walk you through the essential elements, helping you conquer those algebra challenges.

Image: www.pinterest.com
Whether you’re facing a standardized test or simply striving to understand the concepts of algebra, this comprehensive guide will equip you with the tools to succeed. We’ll demystify the world of parent functions and transformations, making them accessible to everyone, regardless of their prior mathematical experience. So, get ready to explore the hidden patterns within equations, and unlock the power of visual representation in algebra.
What are Parent Functions and Transformations?
Imagine a basic blueprint for a house. This blueprint is your parent function, the simplest form of a particular function family. Think of it as the foundation upon which you build various variations. Transformations are like the modifications you make to the blueprint, adjusting the house’s shape, size, and location. The parent function provides a starting point, while transformations determine the final appearance of the function.
Here’s where things get exciting. Transformations involve manipulating the parent function in predictable ways, altering its graph in a controlled manner. Let’s take a closer look at these powerful manipulations.
The Key Transformations: A Detailed Exploration
1. Translations
Imagine sliding a house across a plot of land. That’s essentially what translations do to a function’s graph: shifting it left, right, up, or down without altering its shape.
Here’s how translations are expressed in equations:
- Vertical Translation: Add or subtract a constant c to the parent function.
If c is positive, the graph shifts upwards; if it’s negative, it shifts downwards. For example, y = f(x) + 2 shifts the graph of y = f(x) up by 2 units. - Horizontal Translation: Add or subtract a constant c within the function’s input. If c is positive, the graph shifts left; if it’s negative, it shifts right. For example, y = f(x – 3) shifts the graph of y = f(x) right by 3 units.
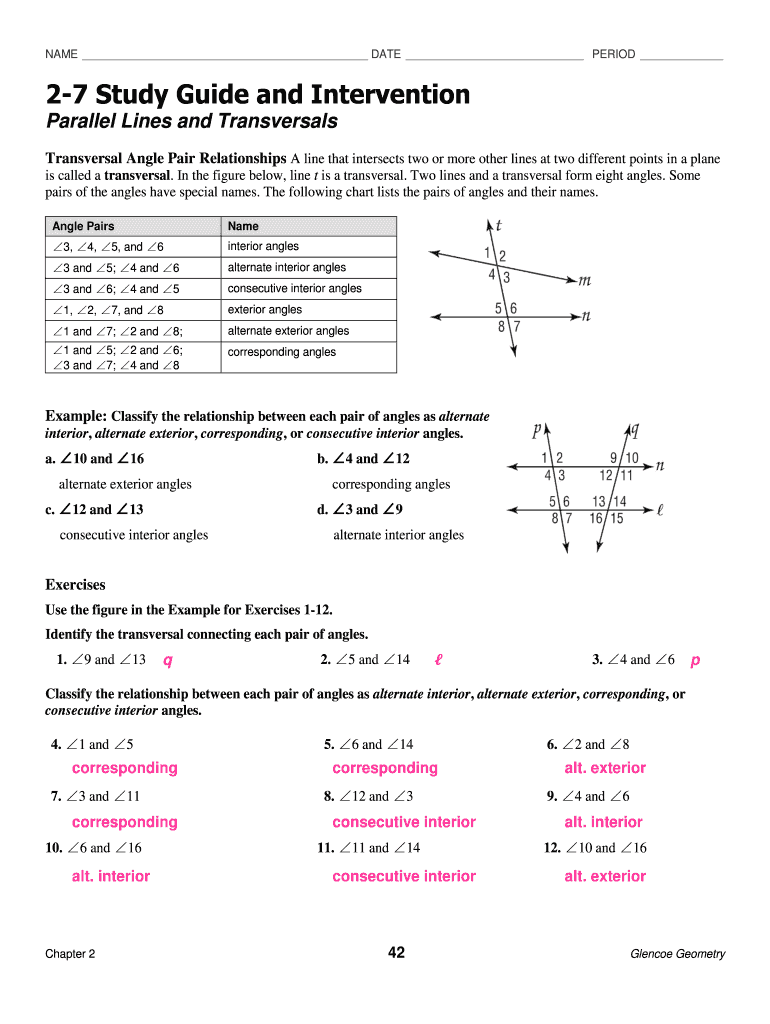
Image: www.pdffiller.com
2. Stretches and Compressions
Want to make your house wider or taller? Stretches and compressions do just that to functions. They change the graph’s shape, either expanding it or condensing it.
- Vertical Stretch/Compression: Multiply the parent function by a constant c. If c is greater than 1, the graph stretches vertically; if c is between 0 and 1, the graph compresses vertically. For example, y = 2f(x) stretches the graph of y = f(x) vertically by a factor of 2.
- Horizontal Stretch/Compression: Multiply the input of the function by a constant c. If c is greater than 1, the graph compresses horizontally; if c is between 0 and 1, the graph stretches horizontally. For example, y = f(3x) compresses the graph of y = f(x) horizontally by a factor of 3.
3. Reflections
Imagine looking at your house in a mirror. Reflections are like that, flipping the function’s graph across an axis.
- Reflection across the x-axis: Multiply the function by -1. For example, y = -f(x) reflects the graph of y = f(x) across the x-axis.
- Reflection across the y-axis: Multiply the input of the function by -1. For example, y = f(-x) reflects the graph of y = f(x) across the y-axis.
Applying Parent Functions and Transformations: Unlocking the Secrets of Equations
Now that you understand the fundamentals of parent functions and transformations, let’s put them into action. Imagine you’re given an equation such as y = -2(x + 1)^2 + 3. From this equation, you can decipher all the changes applied to the parent function.
Let’s break it down:
- Parent Function: The parent function in this case is y = x^2, a basic parabola.
- Horizontal Translation: The term (x + 1) indicates a shift of 1 unit to the left.
- Vertical Stretch: The coefficient -2 signifies a vertical stretch by a factor of 2 and a reflection across the x-axis.
- Vertical Translation: The constant term +3 represents a shift of 3 units upwards.
By understanding the role of each term, we can visualize the entire process of transformation, starting from the parent function and arriving at the final graph. This way, even complex equations become transparent.
Practice Makes Perfect: Tips and Strategies for Success
The best way to solidify your understanding of parent functions and transformations is through consistent practice. Try transforming various parent functions by applying different combinations of stretches, compressions, reflections, and translations.
Here are some strategies to enhance your learning:
- Visual Representation: Graphing functions and transformations will help you develop a strong visual understanding of the concepts.
- Real-World Applications: Think about how transformations are used in everyday scenarios. For example, consider the changing shape of a balloon as it is inflated or the adjustments made to a photograph during editing.
- Practice Problems: Work through a variety of practice problems, focusing on identifying the parent function and each type of transformation. Utilize online resources and textbooks to find plenty of relevant examples.
FAQs: Your Questions Answered!
Q: What is the significance of parent functions in math?
A: Parent functions provide a foundation for understanding various function families and their behavior. By learning the characteristics of parent functions, you can quickly grasp the behavior of their transformed counterparts.
Q: How do transformations impact the domain and range of a function?
A: Transformations can alter the domain and range of a function. For example, a horizontal shift will change the function’s domain, while a vertical shift will affect its range.
Q: Is there a standardized approach to transforming functions?
A: Yes, there is a systematic approach to transforming functions. You can follow the order of operations, applying translations before stretches and compressions.
2 7 Study Guide And Intervention Parent Functions And Transformations
Conclusion
Mastering parent functions and transformations is an essential skill in algebra. This guide has equipped you with the foundational knowledge and strategies to tackle any transformation-related challenge. Remember, practice is key! Apply the tips and techniques discussed to develop confidence and excel in your algebraic journey.
Are you interested in exploring advanced techniques for transforming functions? Let us know in the comments below!






