The world is full of uncertain outcomes. From the flip of a coin to the weather forecast, probability helps us understand and navigate the unpredictable. When it comes to high school math, grasping probability concepts is crucial, especially when preparing for the Common Core Math 2 test. This test often includes problems that require students to apply probability skills to analyze real-world scenarios, and that’s where a solid review of probability concepts comes in handy.
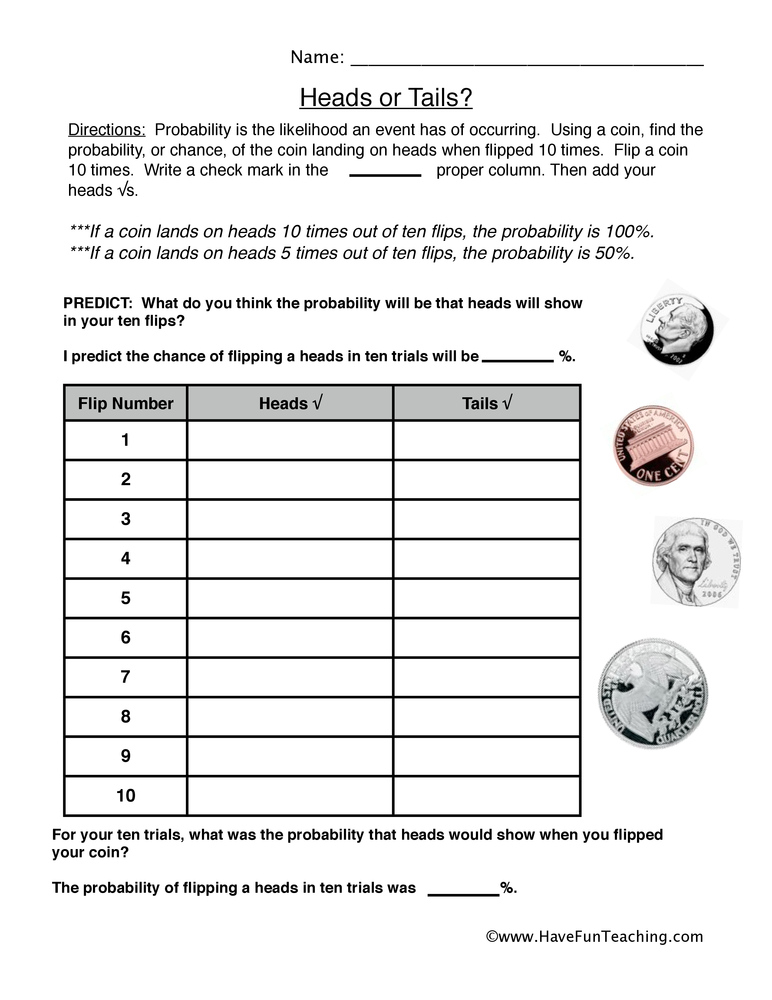
Image: commoncore-worksheets.com
Remember that old saying, “Practice makes perfect”? Well, that’s especially true for probability. Whether you’re studying for the Common Core Math 2 test or simply aiming to sharpen your probability skills, a good review worksheet can make all the difference. This review worksheet focuses on essential probability principles to prepare you for the challenges that the exam might throw your way. It’s a valuable resource for any student who wants to feel confident and prepared to tackle the toughest probability problems and understand the world of chance a little better.
Understanding Probability: The Basics
Probability is a branch of mathematics that deals with the likelihood of events occurring. It quantifies the chance of something happening, expressed as a number between 0 and 1.
Think about it this way: Imagine you’re flipping a coin. There are two possible outcomes: heads or tails. The probability of getting heads is 1/2 (or 50%), because there’s one favorable outcome (heads) and two possible outcomes (heads or tails). The probability of an event is the number of favorable outcomes divided by the total number of possible outcomes.
Key Terms:
- Event: A specific outcome or set of outcomes that we are interested in. For example, getting heads on a coin flip is an event.
- Sample Space: The set of all possible outcomes of an experiment. In the coin flip example, the sample space is heads, tails.
- Probability: The measure of the likelihood of an event occurring. It’s a value between 0 and 1, where 0 represents impossible and 1 represents certain.
Exploring Probability Concepts: From Basics to Advanced

Image: au.edugain.com
1. Basic Probability:
Basic probability focuses on calculating the chances of a single event happening. For instance, if we roll a fair die, what is the probability of rolling a 3? In this case, there’s one favorable outcome (rolling a 3) and six possible outcomes (rolling a 1, 2, 3, 4, 5, or 6). So, the probability of rolling a 3 is 1/6.
2. Compound Events:
Compound events involve the probability of two or more events happening together. Take the example of flipping a coin twice. What is the probability of getting heads on both flips? Here, each flip is an independent event, meaning the outcome of one flip doesn’t affect the outcome of the other flip. To find the probability of both events happening, we multiply the probability of each individual event. So, the probability of getting heads on both flips is (1/2) * (1/2) = 1/4.
3. Conditional Probability:
Conditional probability digs deeper, addressing the likelihood of an event happening given that another event has already occurred. Imagine a bag with three red balls and two blue balls. What’s the probability of picking a red ball, given that you already picked a blue ball and didn’t put it back in the bag? In this case, the total number of outcomes (balls in the bag) has changed. The probability is now 3/4 (three red balls remaining) because the original sample space has been reduced.
4. Independent and Dependent Events:
Understanding the difference between independent and dependent events is crucial. Independent events are not influenced by each other. Rolling a die and flipping a coin are independent events because the outcome of rolling the die doesn’t affect the outcome of the coin flip. Dependent events, on the other hand, are influenced by prior events. Pulling a card from a deck, without replacing it, and then drawing another is dependent because the outcome of the first draw affects the second draw.
Mastering Probability with Practice and Review: Tips for Success
1. Practice Makes Perfect:
The key to mastering probability is consistent practice. Working through problems similar to those on the Common Core Math 2 test will help you build confidence and improve your skills. This includes solving problems that involve different types of probabilities, including conditional probability, independent and dependent events, and compound events.
2. Visualize and Draw Diagrams:
Visualizing probability problems can help make them more understandable. Tree diagrams are extremely useful for illustrating compound events, while Venn diagrams can help visualize conditional probability problems.
3. Engage with Resources:
Besides the Common Core Math 2 Probability Test Review Worksheet 2, there are many online resources and textbooks that can help you reinforce your understanding of probability concepts. Take advantage of interactive tutorials, practice quizzes, and videos that explain probability concepts in a clear and engaging way.
Frequently Asked Questions (FAQs)
**Q: What are some common mistakes students make with probability?**
**A:**
A common mistake is misunderstanding independent and dependent events. Students may incorrectly assume that events are independent when they are actually dependent, or vice versa. Another mistake is forgetting to adjust the sample space for conditional probability problems.
**Q: How can I make sure I understand probability concepts thoroughly?**
**A:** The best way to make sure you grasp probability concepts is by working through diverse practice problems. This lets you apply what you’ve learned in various situations and helps identify any areas that need more attention.
Common Core Math 2 Probability Test Review Worksheet 2
Conclusion
The Common Core Math 2 Probability Test Review Worksheet 2 can be a valuable tool in your probability journey. Mastering probability principles is not just about passing a test; it’s about developing critical thinking skills that apply to real-world situations. By understanding the concepts presented in this worksheet and practicing regularly, you can gain a strong grasp of probability and build confidence in your problem-solving abilities.
Are you ready to conquer the world of probability? Do you have any questions about the topics discussed in this article? Let us know in the comments!






