Picture this: You’re at the beach, building a sandcastle. You diligently pack the sand, hoping it will withstand the tide. You sculpt towers and moats, only to watch them crumble as the waves crash in. Why? The answer lies in the very essence of the sand itself, its density. This seemingly simple concept of density plays a crucial role in our world, shaping everything from the sandcastle’s stability to the design of airplanes. This article will delve into the fascinating world of density, using Unit 1 Worksheet 4 as our guide, and unlock the secrets of how it governs the things around us.

Image: studylib.net
Density might seem like a dry, abstract concept, but it’s anything but. It’s the very foundation of our understanding of materials, and it helps us answer questions like: why does a helium balloon float? Why does a ship made of steel stay afloat on water? Why does a piece of lead feel heavier than a piece of wood of the same size? These are just a few examples of everyday situations where density plays a key role, and understanding its principles can empower you with a new perspective on the world around you.
Understanding the Foundation: Density Defined
At its core, density is a measure of how much mass is packed into a given volume. It’s a simple concept, but it holds immense power in explaining the diverse behaviors of different substances. The formula for density is straightforward: Density (D) = Mass (M) / Volume (V). This means that if you know the mass and volume of an object, you can calculate its density. For example, a block of wood with a mass of 50 grams and a volume of 100 cubic centimeters has a density of 0.5 grams per cubic centimeter.
Exploring Worksheet 4: Practical Applications of Density
Let’s dive into Unit 1 Worksheet 4 and see how these principles are applied in real-world scenarios. The worksheet likely presents a range of problems requiring you to apply the density formula and analyze the relationship between density, mass, and volume. This is where the real fun begins! We’ll dissect a few typical problems to demonstrate the power of density calculations.
Problem 1: Finding the Density of a Solid
Imagine you have a piece of metal, and you need to determine its density. You measure its mass to be 250 grams and its volume to be 50 cubic centimeters. Using the density formula, you divide the mass (250 grams) by the volume (50 cubic centimeters), and you get a density of 5 grams per cubic centimeter. This value gives you a unique identifier for that specific metal, allowing you to compare it to other materials and potentially identify its composition.
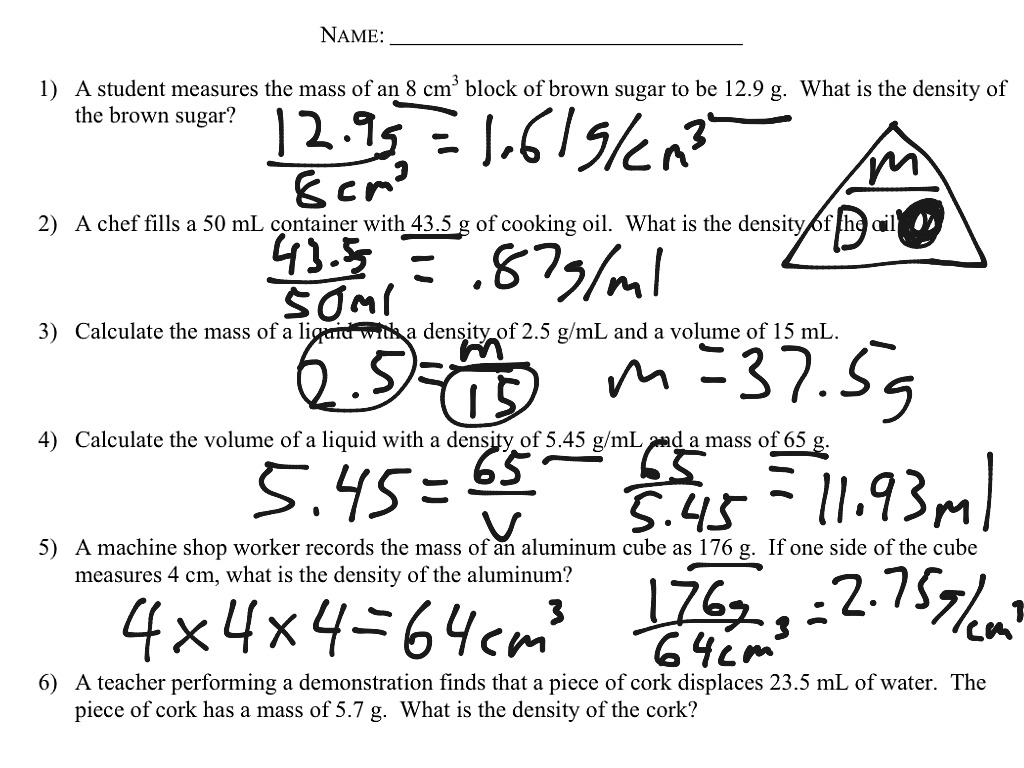
Image: handicraftsied.blogspot.com
Problem 2: Finding the Mass of an Object Given Its Density and Volume
Now, let’s say you know the density of a substance (like water) and the volume of a specific object (like a bucket). Worksheet 4 might ask you to determine the mass of the water in the bucket. Here, you would rearrange the density formula to solve for mass: Mass (M) = Density (D) x Volume (V).
Consider the density of water to be approximately 1 gram per cubic centimeter. If your bucket holds 1000 cubic centimeters of water, its mass would be 1 gram per cubic centimeter x 1000 cubic centimeters, equaling 1000 grams or 1 kilogram. This simple calculation allows you to understand the relationship between volume, density, and mass, making you a more informed citizen of the world.
Problem 3: Finding the Volume of an Object Given Its Density and Mass
Let’s switch gears and consider a scenario where you are given the density of a substance and the mass of an object made from that substance. This is a common situation when dealing with liquids, where you might know the density of a liquid (like oil) and the mass of a certain amount of that liquid. To determine the volume, you rearrange the density formula to solve for volume: Volume (V) = Mass (M) / Density (D).
Let’s say you have 500 grams of oil, and you know that the density of oil is approximately 0.9 grams per cubic centimeter. You can calculate the volume by dividing the mass (500 grams) by the density (0.9 grams per cubic centimeter), resulting in a volume of 555.56 cubic centimeters.
Beyond the Worksheet: Real-World Density Applications
The principles you learn in Unit 1 Worksheet 4 aren’t confined to textbook problems. They have profound implications for our daily lives and even influence the advancement of various technologies.
1. Engineering Marvels: Density in Aircraft Design
The Wright Brothers, who achieved the first successful airplane flights, understood the importance of density. For an aircraft to take flight, it needs to be lighter than the air it displaces. This is where density comes into play. Engineers meticulously design aircraft with materials that have low densities, such as aluminum and composites, while ensuring that they are strong enough to withstand the stresses of flight.
2. The Buoyancy of Ships: A Tale of Density
Have you ever wondered how massive ships, made of steel, can stay afloat on water? It’s all down to density. Ships are designed to displace more water than their own weight. The water they displace creates an upward force called buoyancy, which counteracts gravity and keeps the ship afloat. The denser the material used in a ship, the more weight it will displace, necessitating a larger hull. It’s a fascinating example of how density dictates the behavior of objects in fluids.
3. The Science of Food: Density in Cooking
Density plays a crucial role in cooking, too. When you bake a cake, for example, the batter’s density determines how evenly it will rise and cook. A denser batter will produce a heavier cake, while a lighter batter will result in a fluffier cake. The density of ingredients like flour, sugar, and eggs will influence the overall texture and consistency of your culinary creations.
Expert Insights: Unlocking Density’s Potential
Mastering the concepts of density can open up a world of possibilities. Here are some insights from experts that can guide you on this journey:
“Be a Density Detective:” Dr. Sarah Jones, Physics Professor
Dr. Jones suggests a fun experiment: “Take a variety of objects around your house, such as a book, a bottle of water, a piece of metal, and a piece of wood. Use a scale to measure their masses and measure their volumes using displacement methods. Calculate their densities and compare them. You’ll be amazed at how varied the densities of everyday objects can be.”
“Think Beyond the Basics:” Dr. David Miller, Materials Science Engineer
Dr. Miller encourages you to think about the practical implications of density: “Understanding density helps us answer important questions in materials science. For example, when designing a bridge, we need to choose materials that are strong enough to support the weight of vehicles but also lightweight enough to be easily constructed. Density is a key factor in making these decisions.”
Unit 1 Worksheet 4 Applied Density Problems
Conclusion: Embracing the Density of the World Around Us
Density might seem like a simple concept, but it underpins a vast array of phenomena in our world. Understanding it empowers you to approach everyday situations with a deeper understanding of why things behave the way they do. Unit 1 Worksheet 4 serves as a stepping stone, guiding you through practical applications of density calculations. Beyond the worksheet, remember to embrace the vastness of density’s influence—from sandcastles that crumble to towering skyscrapers that reach for the sky. Density is the unseen force that shapes the world around us, and by understanding its principles, you can become a more informed and empowered individual.






