Have you ever wondered how architects design buildings that stand strong and steady? Or how engineers construct bridges that can withstand the weight of tons of traffic? The answer lies in the fascinating world of geometry, where shapes and their properties play a crucial role. In this journey, we’ll delve into one specific geometric figure – the parallelogram – and explore its properties through the lens of a common Geometry Worksheet, specifically focusing on Worksheet 6.2. Get ready to unlock the secrets of parallelograms and gain a deeper understanding of their real-world applications.
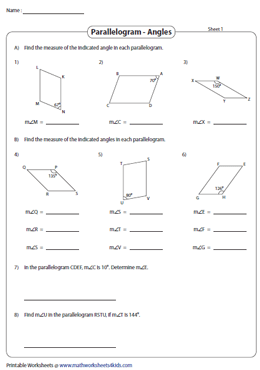
Image: www.mathworksheets4kids.com
Geometry Worksheet 6.2, usually assigned in a high school geometry course, delves into the properties and characteristics of parallelograms. This worksheet often includes various problems that require students to apply theorems and concepts pertaining to parallelograms. It serves as a stepping stone for students to master the fundamental ideas related to parallelograms and their applications in various areas of geometry.
Understanding Parallelograms
Definition and Properties
A parallelogram is a quadrilateral (a four-sided polygon) with two pairs of parallel sides. These parallel sides are equal in length, forming a unique set of characteristics that define this specific shape. The properties of a parallelogram are crucial to solving problems related to this shape.
- Opposite sides are parallel and equal in length: This fundamental property is the defining feature of a parallelogram.
- Opposite angles are equal: The angles opposite each other within the parallelogram are congruent.
- Consecutive angles are supplementary: If two angles share a side, their sum is always 180 degrees.
- Diagonals bisect each other: When the two diagonals of a parallelogram intersect, they cut each other in half.
Real-World Applications of Parallelograms
Parallelograms are more than just geometric shapes; they appear in various real-world scenarios:
- Architecture: From windows to floor tiles, parallelograms are employed to create unique architectural designs.
- Engineering: The stability of structures like bridges and buildings often relies on the sturdiness of parallelogram-shaped components.
- Art and Design: Artists and designers frequently utilize parallelograms to create dynamic compositions and patterns.
- Nature: Even in the natural world, parallelograms are observed in honeycombs built by bees and the patterns on leaves of certain plants.
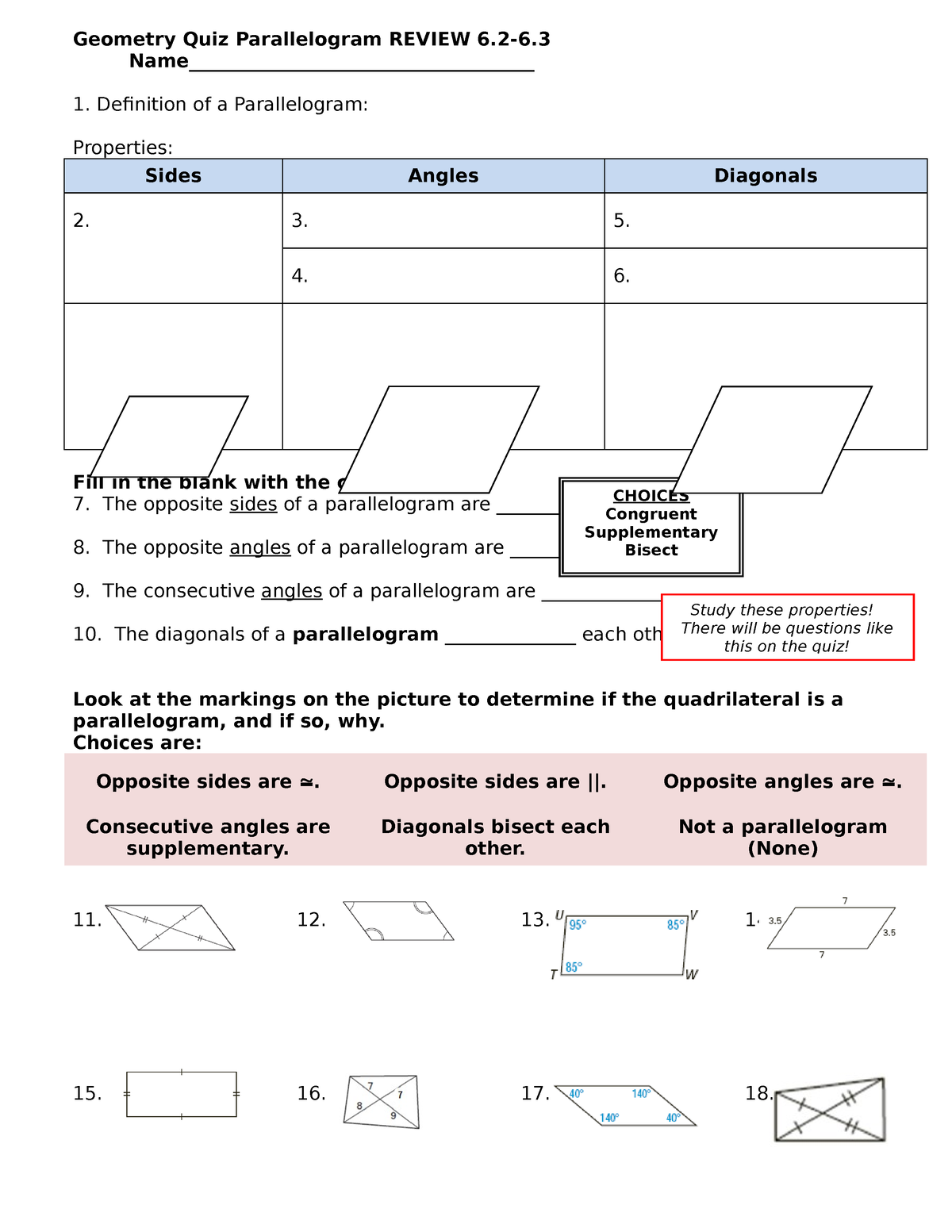
Image: www.studocu.com
Solving Problems with Worksheet 6.2
Types of Problems Encountered
Geometry Worksheet 6.2 usually presents students with a variety of problems related to parallelograms. These problems might involve:
- Finding missing side lengths or angle measures: By applying the properties of parallelograms, students can determine unknown values based on given information.
- Proving that a given quadrilateral is a parallelogram: This requires applying the properties of parallelograms as well as other geometric principles.
- Using coordinate geometry to prove parallelogram properties: Students may be asked to use the coordinate plane to demonstrate the relationships between points, sides, and diagonals of parallelograms.
Key Concepts and Strategies
To tackle problems in Worksheet 6.2 successfully, students need to grasp certain key concepts:
- Understanding the definitions and properties of parallelograms: This is the foundational knowledge required to solve any problem related to parallelograms.
- Applying geometric theorems and postulates: Many problems will necessitate the application of established geometric principles.
- Using algebraic techniques: Some problems may involve solving equations or inequalities to determine unknown values.
- Developing logical reasoning and deductive skills: Geometry problems often require students to combine evidence and draw logical conclusions.
Examples of Problems and Solutions
Here’s a sample problem found in Worksheet 6.2 that illustrates the application of parallelogram properties:
Problem: In parallelogram ABCD, AB = 10 cm, AD = 8 cm, and angle A measures 60 degrees. Find the length of diagonal BD.
Solution:
- Draw a diagram: Sketch parallelogram ABCD, labeling sides and angles according to the given information.
- Apply properties: We know that opposite sides of a parallelogram are equal, so BC = AD = 8 cm. Also, consecutive angles of a parallelogram are supplementary, so angle B = 180 – 60 = 120 degrees.
- Use the Law of Cosines: Triangle ABD is a triangle with known side lengths and one angle. We can use the Law of Cosines to find the length of diagonal BD: BD² = AB² + AD² – 2(AB)(AD)cos(A).
- Solve for BD: Substituting the given values, BD² = 10² + 8² – 2(10)(8)cos(60). Simplifying the equation, we get BD² = 100 + 64 – 80 = 84. Therefore, BD = √84 ≈ 9.17 cm.
This example demonstrates how applying the properties of parallelograms and basic trigonometric knowledge can help solve problems related to these geometric figures.
Beyond Worksheet 6.2: Exploring Parallelograms Further
Exploring Advanced Concepts
While Worksheet 6.2 covers fundamental concepts of parallelograms, there’s a vast world of knowledge beyond its scope. Some of these advanced concepts include:
- Special types of parallelograms: There are specific types of parallelograms like rectangles, squares, and rhombuses that have additional properties beyond the general parallelogram.
- Area and perimeter formulas: Specific formulas exist for calculating the area and perimeter of parallelograms, which are more complex than those for basic quadrilaterals.
- Parallelograms in three-dimensional space: Parallelograms can also be extended to three dimensions. Explore concepts like parallelepipeds and understand how these shapes form the basis of many geometric objects.
Real-Life Examples and Applications
To further appreciate the importance of parallelograms, consider their applications in various fields:
- Architecture: Imagine the designs of modern skyscrapers – their façades often incorporate parallelogram shapes for aesthetic appeal and structural integrity.
- Engineering: From suspension bridges to truss designs, parallelograms are used extensively in structural engineering due to their strength and stability.
- Art and Design: The world of art is filled with examples of parallelogram-based compositions. Consider famous works like “The Last Supper” by Leonardo da Vinci, where the arrangement of figures is based on a parallelogram.
Geometry Worksheet 6.2 Parallelograms Answer Key
Conclusion
Completing Geometry Worksheet 6.2 is more than just a school assignment; It opens a door to the world of geometry and its practical applications. Understanding the properties of parallelograms unlocks a deeper appreciation for how shapes contribute to the structures, designs, and patterns that surround us. As you continue to explore the fascinating realm of geometry, remember the fundamental concepts learned from Worksheet 6.2 – they serve as building blocks for more complex mathematical endeavors. Don’t hesitate to delve deeper, explore advanced concepts, and discover how parallelograms shape the world around us!






